Music is really just orderly vibrations: in the air, in instrument bodies, in speaker cones, in tiny hairs in your inner ear, in electromagnetic fields in wires, in patterns of neurons firing in your brain. If you understand the math behind these vibrations, it can help you understand how music works. Surprisingly, it can also help you understand quantum mechanics and the fundamental structure of the universe. No joke! Albert Einstein himself used music theory to guide his investigation into the vibrations of the subatomic world. Einstein’s preferred tool for musical investigation was the violin, but any instrument will do.
First of all, to get your feet under you, here’s a video by the delightful Vi Hart explaining the basic physics of sound:
Music theory and wave mechanics
I’m going to talk you through the relationship between wave mechanics and music theory using the low E string on the guitar. If you have one handy, grab it and follow along. The experiment is easier on electric guitar with the amp turned up, but it works fine on acoustic as long as the room is quiet. If you don’t have a stringed instrument available, there are a bunch of Youtube videos you can watch, including this one, this one, and this one.
Vibrational modes
When you see a cartoon of a plucked string, it shows vibration lines spanning the full length of the string, implying that the middle of the string is swinging to and fro. Real strings vibrate this way, but they also vibrate in more complex ways too. Strings can vibrate in halves, with one half bowing this way while the other bows that way. Strings can also vibrate in thirds, the middle third bowed this way while the outer thirds bow that way, and vice versa. They simultaneously vibrate in fourths, fifths, sixths, sevenths and so on, in smaller and smaller parts, in theory going all the way to infinity.
When you pluck a guitar string, its movements are a combination of all these patterns of vibration combined together.
Resonant frequencies
Guitar strings vibrate really fast, so it’s hard to see all the different vibrational patterns firsthand. Jumpropes work better. Imagine that you and I are holding a big long jumprope. I’m holding one end still, and you’re waving your end up and down. If you wave the rope up and down at a certain frequency, the jumprope’s resonant frequency, you can make it vibrate along its entire length. If you wave it up and down twice that fast, you can make it vibrate in halves. Waving it three times faster makes the rope vibrate in thirds. Waving four times faster makes it vibrate in fourths. The pattern continues indefinitely, with more and more effort required on your part to make the rope vibrate in more and more sections.
If you could organize a whole bunch of people to carefully all shake the rope at once, each person shaking at a different multiple of the rope’s resonant frequency, the rich blend of movements would produce a perfect slow-mo replica of a guitar string’s vibrational pattern. This would be hard to do in real life, so you can use computer animation to assist your visualizing. See also this amazing stroboscopic video of an upright bassist.
Harmonics
The different multiples of the jumprope’s resonant frequency are called harmonics. If the word reminds you of harmony, it should. The rate of shaking that produces the basic cartoon-style full-length vibration is the fundamental. When each harmonic is a whole-number multiple of the fundamental frequency, and the vibration is fast enough to agitate the air audibly, you hear a pleasantly musical sound.
The harmonics don’t have to be perfectly aligned to whole-number ratios. If they’re random, the sound you hear is abrasive, or dull, or harsh, or strange. If you blend together the simple whole-number multiples of the resonant frequency with more complex or random multiples, you get sounds that are musical but otherworldly, like bells, gongs and the synthesizers in hip-hop and techno.
Tonal instruments like the guitar has been designed to maximize the rational, whole-number harmonics and to minimize the random and irrational ones. When you use distortion on an electric guitar, it compresses the sound and makes it exceptionally easy to hear the harmonics. Jimi Hendrix was a pioneer of the use of electric guitar harmonics.
The harmonics of the guitar’s E string
When you pluck the low E string on a guitar, the loudest sound you hear is the fundamental tone as the string vibrates along its entire length. If it’s in tune, the E string’s middle crosses its relaxed position about 165 times each second. This is the pitch known to western music theory as E2. It’s the second-lowest E on a piano.
The guitar’s E string is also vibrating in halves, each half crossing the relaxed position 330 times each second, twice the fundamental frequency, to produce the note E3. You can hear this harmonic more clearly if you silence the fundamental by deadening the string lightly with a fingertip right above the twelfth fret, the string’s halfway point, as you pluck it.
Your ear-brain system is very adept at sussing out when one frequency is twice another frequency, even if they’re being produced simultaneously by the same vibrating string. We experience this pattern-detection ability as our sense of harmony. Nearly everyone can identify E2 and E3 as being “same” pitch, even though one is “higher” than the other. The western musical name for a two to one ratio of frequencies is an octave. What music theory calls “octave equivalence” is your experience of frequencies related by powers of two as being, in a sense, “the same.” The ability to detect octaves appears to be a human universal, and apes and monkeys can detect octaves too.
There are a lot of octaves of E hidden in the string’s quieter sub-vibrations. You can make the string vibrate in quarters by plucking it while touching it lightly above the fifth fret. As the string vibrates, each quarter crosses the relaxed position 660 times per second, to produce the note E4. As the string vibrates in eighths, each section produces a very quiet E5. As the string vibrates in sixteenths, each section produces an even quieter E6. If you plucked a perfect string on a perfect guitar, you’d hear every E up past the limits of your pitch perception, each one produced by a power-of-two multiple of the fundamental E2 frequency. These harmonics are reinforced by sympathetic vibrations from the guitar’s other E string, whose fundamental is E4. As the high E mutually agitates with the low E, it contributes its own multiples of eighty-two and a half to the mix.
The major triad emerges from the overtone series
All those ghostly bright E’s hovering above the fundamental frequency are only the beginning of the complexity hidden in an ordinary guitar string. To hear the string vibrating in thirds, touch it lightly above the seventh fret while plucking it. As the E string vibrates, each third crosses the relaxed position two hundred forty-seven times each second. This frequency is the pitch B3. The ratio between B3 and E3 is known to western music theory as the perfect fifth. The ratio of B3 to E4 is a perfect fourth. Like the octave, these ratios are heard across nearly every world culture. It’s no accident that the guitar has a string tuned to B3. Its sympathetic vibrations as you play the E strings are part of the instrument’s distinctive sound.
As the E string vibrates in fifths, each fifth crosses the relaxed position 824 times per second, producing the note G#4. You can hear this harmonic by lightly touching the string between the third and fourth frets and plucking hard. The ratio of frequencies between G#4 and E4 is a major third. After octaves, fifths and fourths, major thirds are the next most common interval in western music, and in many other musical cultures as well.
The ratio of G#4 to B4 is a minor third, the frequently-heard “sad” counterpart to the “happy” major third. Not every culture ascribes these emotional qualities to these ratios in every context, but most of the time, most western listeners experience major thirds as happy and minor thirds as sad.
Higher harmonics, more complex intervals
As you continue along the harmonic series, the ratios get more complex and the sounds get more mysterious. As the string vibrates in sevenths, it produces a very high-pitched sound close to a D. The interval between E and D is a flat or minor seventh. As the string vibrates in ninths, you get a note very close to F#, a natural second above E. As the string vibrates in elevenths, you get something close to Bb or A#, a tritone above E. Tritones are a key ingredient in blues, jazz, rock and their musical descendants.
The chord hidden in a single note
With all of its harmonics, the note E on a guitar is more like a richly complex chord. You’re not just hearing E in several different octaves. You’re hearing a quiet B, an even quieter G#, a faint D and a fainter F# and a barely perceptible A#. You’re hearing the chord that jazz musicians call E9#11, whose pitches comprise the acoustic scale. Jazz musicians call it the Lydian dominant mode; western classical nicknames it the Bartók scale.
And this is all from a single note. When you play two notes at a time, or three or four, you get even more complex harmonic interaction from all the overtones.
Every vibrating physical system can have harmonics
The cool thing about all of this harmonic business is that it isn’t specific to guitar strings or air or eardrums. Anything that vibrates steadily can produce harmonics: your throat, drum heads, gongs, the mouthpieces and bodies of wind instruments, organ pipes, bones, hollow logs, shopping carts, garbage cans, ladders, water glasses, crystals, even single molecules and atoms.
Most rigid material objects have a resonant frequency. You can shatter a wine glass by singing its resonant frequency.
Bridges have resonant frequencies too. High school science teachers love to show films of the Tacoma Narrows Bridge as the wind shakes it at its resonant frequency until it collapses.
Quantum particles have harmonics too
If you want to understand the fundamental structure of matter, you need to know about harmonics. All those pictures you see of marble-like electrons orbiting a nucleus like moons orbiting a little planet are totally wrong. In reality, electrons are organized in atoms by the harmonics of the electron field. Here’s a blog post explaining this concept in detail. Who says music is frivolous?

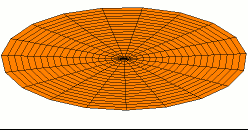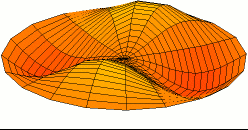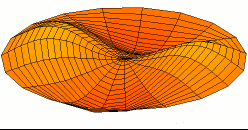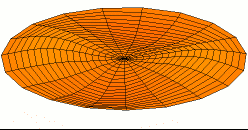

Came here via Quora.
The low E string vibrates at ~82.4 Hz. That means it would cross the middle position twice per cycle, or ~165 times, not 82.5 times. Or am I missing something?
(If I am right, I think you’d have to double the values of all the middle-crossings you have mentioned)
True! Correcting that now.
Heh, I found this while trying to find out why my guitar makes a weird sound but only on one fret. But as I’m a science kind of guy I was intrigued…very good post, and I enjoy trying to comprehend everything I can see as particles emitting waves :D And usually stop when my head hurts.